25 Section Summary

In this section of modern algebra, we discussed groups, rings & fields, and rings of polynomials. Again, it is the author’s regret, owing to the page restriction, there are so much more to be discussed. The list of a few missing items are as follows:

• In groups, the theorem on homomorphic properties that a homomorphic mapping preserves the identity element, inverses, and subgroups; applying the notion of to matrix algebra – for example, 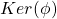is in fact the null space of 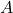in matrix algebra; an alternative strategy to show isomorphism using the concept of kernel; the theorem on equivalent expressions of a normal subgroup; and permutations
• In rings and fields, the famous Fermat’s and Euler’s theorems; and the expansion of an integral domain into a field of quotients
• In rings of polynomials, important theorems on reducibility and irreducibility; Eisenstein criterion on the irreducibility over 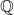; and cytoclomic polynomials.

Still, after familiarizing yourself with the essentials provided in this summary, the reader would be tuned to be able to deepen his or her understanding in modern algebra.