P-1. Physical Quantities, Units, and Measurements
Physics Department of Douglas College; OpenStax; and Joey Wu
Summary
- Understand the definitions of quantity, fundamental quantity, and derived quantity.
- Explain the most common prefixes in the SI units and be able to write them in scientific notation

The world around us is full of things we can measure, from very small to very large. Think about the size of a grain of sand compared to the height of a tall building, or the weight of a feather compared to the weight of an elephant. To understand and describe these different sizes and amounts, we use numbers and units.
When we talk about a physical quantity, we’re referring to something we can measure or calculate. For example, we can measure how tall someone is or how long it takes to run across a playground. We can also calculate things like how fast someone is running by using other measurements we’ve made. Every measurement provides three kinds of information:
- the size or magnitude of the measurement (a number),
- a standard of comparison for the measurement (a unit), and
- an indication of the uncertainty of the measurement.
While the number and unit are explicitly represented when a quantity is written, the uncertainty is an aspect of the measurement result that is more implicitly represented and will be discussed later.
The number in the measurement can be represented in different ways, including decimal form and scientific notation. (Scientific notation is also known as exponential notation.) For example, the maximum takeoff weight of a Boeing 777-200ER airliner is 298,000 kilograms, which can also be written as 2.98 x 105 kg. The mass of the average mosquito is about 0.0000025 kilograms, which can be written as 2.5 x 10−6 kg.
When we measure the physical quantities, we use units. Units are agreed-upon standard amounts that everyone understands. For instance, we might measure someone’s height in centimeters or inches, or measure the length of a football field in meters or yards. Using these standard units helps everyone understand and compare measurements.
Without units, a number can be meaningless, confusing, or possibly life threatening. Suppose a doctor prescribes phenobarbital to control a patient’s seizures and states a dosage of “100” without specifying units. Not only will this be confusing to the medical professional giving the dose, but the consequences can be dire: 100 mg given three times per day can be effective as an anticonvulsant, but a single dose of 100 g is more than 10 times the lethal amount.
The system of units called the metric system. The most common units in this system are meters for length, kilograms for mass (which is similar to weight), and seconds for time. By using these standard units, scientists (and students!) all over the world can share and understand each other’s measurements.
In the world of measurement, there are two main systems of units that people use: the metric system and the customary system. The metric system, also called SI units (which stands for International System of Units in English; the acronym “SI” is derived from the French Système International), is used by most countries around the world and by scientists everywhere. It includes units like meters, grams, and liters. The customary system, sometimes called English units or imperial units, is mainly used in the United States for everyday measurements. This system includes units like inches, pounds, and cups.
In our science classes, we’ll focus on the metric system because it’s what scientists use and it’s easier to work with. The metric system uses units that are all related by factors of 10, which makes converting between them simpler. For example, there are 100 centimeters in a meter, and 1000 meters in a kilometer. This consistency makes the metric system very useful for scientific measurements and calculations.
Here’s an interesting story that shows why using the same measurement system is so important:
In 1999, NASA (the US space agency) sent a spacecraft called the Mars Climate Orbiter to study Mars. It was supposed to orbit around Mars and send back information about the planet’s weather. But something went wrong, and the spacecraft crashed into Mars instead of orbiting it!
What happened? It turned out that two teams working on the project were using different measurement systems. One team was using metric units (like meters and kilometers), while the other was using customary units (like feet and miles). This meant that when they shared information, they were misunderstanding each other’s numbers.
Because of this mix-up, the spacecraft got too close to Mars and burned up in its atmosphere. This mistake cost $125 million!
This story shows us why it’s so important for scientists to agree on which measurement system to use. It also reminds us to always check our units when we’re doing math and science!
If you’re interested in the story, click https://sma.nasa.gov/docs/default-source/safety-messages/safetymessage-2009-08-01-themarsclimateorbitermishap.pdf?sfvrsn=eaa1ef8_4
SI Units: Fundamental and Derived Units
The metric system, which we use in science, is managed by an international organization in France. This organization makes sure that everyone around the world agrees on how to define and use metric units. Table 1.1 below shows the seven basic metric units. These are called the fundamental units because they’re the building blocks for measuring many things in science:
Base Units of the SI System |
||
|
Property Measured |
Name of Unit |
Symbol of Unit |
|
length |
meter |
m |
|
mass |
kilogram |
kg |
|
time |
second |
s |
|
temperature |
kelvin |
K |
|
electric current |
ampere |
A |
|
amount of substance |
mole |
mol |
|
luminous intensity |
candela |
cd |
Table 1.1
In this course, we will mainly focus on three basic metric units: Length (meter), Mass (Kilogram), and Time (Seconds).
These fundamental units are special because we define them based on how we measure them. For example, we define a meter as the distance light travels in a tiny fraction of a second. There are seven fundamental units in the metric system. In this course, we will mainly focus on three of them, as listed in Table 1.
Units of Time, Length, and Mass: The Second, Meter, and Kilogram
The Second
The second is our unit for measuring time. It’s the time it takes for something very tiny (an atom) to vibrate 9,192,631,770 times! Scientists chose this definition because it’s very precise and doesn’t change. Before this, people used to define a second based on how long it takes the Earth to spin around once, but that wasn’t as accurate because the Earth’s rotation is actually slowing down very gradually.
The Meter
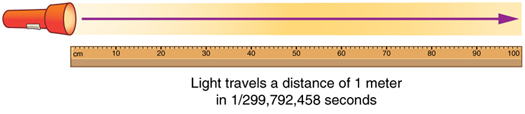
The meter is our unit for measuring length. Today, we define a meter as the distance light travels in about 1/300,000,000 of a second. That’s super fast! Scientists chose this definition because the speed of light is always the same, so it gives us a very accurate way to measure distance.
Fun fact: Before this, people tried defining the meter in different ways. They once said it was one ten-millionth of the distance from the North Pole to the equator. Later, they made a special metal bar and said the meter was the length between two lines on that bar. But using light is much more precise!
The Kilogram
The kilogram is our unit for measuring mass. For a long time, the kilogram was defined by a special metal cylinder kept in France. All other weights in the world were based on this one cylinder! In 2019, scientists redefined the kilogram using a fundamental constant of nature called the Planck constant. This constant relates a particle’s energy to its frequency, and it’s always the same everywhere in the universe.
The new definition involves a special device called the Kibble balance, which uses electromagnetic forces to balance the weight of a mass. By relating this balance to the Planck constant, scientists can now define the kilogram without relying on a physical object. This new definition is more stable and precise than the old method of using a physical cylinder. It ensures that the kilogram will remain constant over time and can be reproduced anywhere in the world using the right equipment.
While the details of this process are complex, the important thing for us to understand is that scientists are always working to make our measurements more accurate and reliable. This new definition of the kilogram is a great example of how science is always improving and refining our understanding of the world.
Why are these definitions so important? Scientists need very precise measurements to do their work. By defining our basic units (second, meter, and kilogram) in these accurate ways, we make sure that everyone around the world is measuring things in exactly the same way. This helps scientists work together and trust each other’s results, no matter where they are in the world.
In our course, we’ll use these units to measure all sorts of things related to motion, force, and energy. Everything we measure will be based on combinations of length, mass, and time!
The Metric System: A Simple Way to Measure Big and Small Things
SI units are part of the metric system. The metric system is convenient for scientific and engineering calculations because the units are categorized by factors of 10. The table below gives metric prefixes and symbols used to denote various factors of 10.
The metric system, which includes our SI units, is great for science because it uses a simple pattern based on the number 10. This makes it easy to convert between different sizes of units.
Here’s why the metric system is so handy:
- Easy Conversions: In the metric system, going from one unit to another always involves multiplying or dividing by 10, 100, 1000, and so on. For example:
- There are 100 centimeters in a meter
- There are 1000 meters in a kilometer. This is much simpler than the customary system used in the U.S., where there are 12 inches in a foot and 5280 feet in a mile!
- Prefixes for Big and Small: The metric system uses special prefixes to show very big or very small measurements. For example:
- “Kilo-” means 1000 times bigger (like in kilometer)
- “Centi-” means 100 times smaller (like in centimeter)
- “Milli-” means 1000 times smaller (like in millimeter)
When we’re dealing with very big or very small numbers in science, we often use something called “order of magnitude.” It is defined as the scale of a value expressed in the metric system. This is a way to describe how big or small something is using powers of 10. It’s like a quick way to estimate size.
Here’s how it works: Each time you multiply by 10, you go up one order of magnitude; Each time you divide by 10, you go down one order of magnitude. For example, 10 is one order magnitude bigger than 1, 100 is two orders of magnitude bigger than 1, 1000 is three orders of magnitude bigger than 1. This is really useful when we’re comparing things that are very different in size. For instance:
- The width of an atom is about 0.0000000001 meters (10^-10 meters)
- The width of a human hair is about 0.0001 meters (10^-4 meters)
- The height of a person is about 1 meter (10^0 meters)
- The diameter of the Earth is about 10,000,000 meters (10^7 meters)
Using orders of magnitude, we can say the Earth is about 17 orders of magnitude wider than an atom! This helps us understand just how much bigger the Earth is compared to an atom.
In your classroom, you can use this concept to help students grasp the vast differences in size between things they study in science, from tiny atoms to huge planets. It’s a great way to build their understanding of scale and proportion, which are important skills in science and math.
| Prefix | Symbol | Value | Example (some are approximate) | |||
|---|---|---|---|---|---|---|
| exa | E | 1018 | exameter | Em | 1018 m | distance light travels in a century |
| peta | P | 1015 | petasecond | Ps | 1015 s | 30 million years |
| tera | T | 1012 | terawatt | TW | 1012 W | powerful laser output |
| giga | G | 109 | gigahertz | GHz | 109 Hz | a microwave frequency |
| mega | M | 106 | megacurie | MCi | 106 Ci | high radioactivity |
| kilo | k | 103 | kilometer | km | 103 m | about 6/10 mile |
| hecto | h | 102 | hectoliter | hL | 102 L | 26 gallons |
| deka | da | 101 | dekagram | dag | 101 g | teaspoon of butter |
| — | — | 100
(=1) |
||||
| deci | d | 10-1 | deciliter | dL | 10-1 L | less than half a soda |
| centi | c | 10-2 | centimeter | cm | 10-2 m | fingertip thickness |
| milli | m | 10-3 | millimeter | mm | 10-3 m | flea at its shoulders |
| micro | µ | 10-6 | micrometer | µm | 10-6 m | detail in microscope |
| nano | n | 10-9 | nanogram | ng | 10-9 g | small speck of dust |
| pico | p | 10-12 | picofarad | pF | 10-12 F | small capacitor in radio |
| femto | f | 10-15 | femtometer | fm | 10-15 m | size of a proton |
| atto | a | 10-18 | attosecond | as | 10-18 s | time light crosses an atom |
| Table 2. Metric Prefixes for Powers of 10 and their Symbols. | ||||||
Known Ranges of Length, Mass, and Time
The vastness of the universe and the breadth over which physics applies are illustrated by the wide range of examples of known lengths, masses, and times show in the table above. Examination of this table will give you some feeling for the range of possible topics and numerical values.

Unit Conversion
Sometimes, we need to change from one unit to another. This is called unit conversion, and it’s an important skill in science and everyday life. In many real-world scenarios, we might need to convert recipes from metric to customary units, or understand distances in different units when reading maps or travel information. Unit conversion also reinforces multiplication, division, and working with fractions, thus helping us build mathematical skills.
Let us consider a simple example of how to convert units. Let us say that we want to convert 80 meters (m) to kilometers (km).
The first thing to do is to list the units that you have and the units that you want to convert to. In this case, we have units in meters and we want to convert to kilometers.
Next, we need to determine a conversion factor relating meters to kilometers. A conversion factor is a ratio expressing how many of one unit are equal to another unit. For example, there are 12 inches in 1 foot, 100 centimeters in 1 meter, 60 seconds in 1 minute, and so on. In this case, we know that there are 1,000 meters in 1 kilometer.
Now we can set up our unit conversion. We will write the units that we have and then multiply them by the conversion factor so that the units cancel out, as shown:
Note that the unwanted m unit cancels, leaving only the desired km unit. You can use this method to convert between any types of unit.
Click here to go the Back Matter Appendices. Appendix Useful Information for a more complete list of conversion factors.
| Lengths in meters | Masses in kilograms (more precise values in parentheses) | Times in seconds (more precise values in parentheses) | |||
|---|---|---|---|---|---|
| 10−18 | Present experimental limit to smallest observable detail | 10−30 | Mass of an electron (9.11×10−31 kg) | 10−23 | Time for light to cross a proton |
| 10−15 | Diameter of a proton | 10−27 | Mass of a hydrogen atom (1.67×10−27 kg) | 10−22 | Mean life of an extremely unstable nucleus |
| 10−14 | Diameter of a uranium nucleus | 10−15 | Mass of a bacterium | 10−15 | Time for one oscillation of visible light |
| 10−10 | Diameter of a hydrogen atom | 10−5 | Mass of a mosquito | 10−13 | Time for one vibration of an atom in a solid |
| 10−8 | Thickness of membranes in cells of living organisms | 10−2 | Mass of a hummingbird | 10−8 | Time for one oscillation of an FM radio wave |
| 10−6 | Wavelength of visible light | 1 | Mass of a liter of water (about a quart) | 10−3 | Duration of a nerve impulse |
| 10−3 | Size of a grain of sand | 102 | Mass of a person | 1 | Time for one heartbeat |
| 1 | Height of a 4-year-old child | 103 | Mass of a car | 105 | One day (8.64×104 s) |
| 102 | Length of a football field | 108 | Mass of a large ship | 107 | One year (y) (3.16×107 s) |
| 104 | Greatest ocean depth | 1012 | Mass of a large iceberg | 109 | About half the life expectancy of a human |
| 107 | Diameter of the Earth | 1015 | Mass of the nucleus of a comet | 1011 | Recorded history |
| 1011 | Distance from the Earth to the Sun | 1023 | Mass of the Moon (7.35×1022 kg) | 1017 | Age of the Earth |
| 1016 | Distance traveled by light in 1 year (a light year) | 1025 | Mass of the Earth (5.97×1024 kg) | 1018 | Age of the universe |
| 1021 | Diameter of the Milky Way galaxy | 1030 | Mass of the Sun (1.99×1030 kg) | ||
| 1022 | Distance from the Earth to the nearest large galaxy (Andromeda) | 1042 | Mass of the Milky Way galaxy (current upper limit) | ||
| 1026 | Distance from the Earth to the edges of the known universe | 1053 | Mass of the known universe (current upper limit) | ||
| Table 3. Approximate Values of Length, Mass, and Time. | |||||
NONSTANDARD UNITS
While there are numerous types of units that we are all familiar with, there are others that are much more obscure. For example, a firkin is a unit of volume that was once used to measure beer. One firkin equals about 34 liters. To learn more about nonstandard units, use a dictionary or encyclopedia to research different “weights and measures.” Take note of any unusual units, such as a barleycorn, that are not listed in the text. Think about how the unit is defined and state its relationship to SI units.
Summary
- Physical quantities are a characteristic or property of an object that can be measured or calculated from other measurements.
- Units are standards for expressing and comparing the measurement of physical quantities. All units can be expressed as combinations of fundamental units.
- The four fundamental units we will use in this text are the meter (for length), the kilogram (for mass), the second (for time), and the ampere (for electric current). These units are part of the metric system, which uses powers of 10 to relate quantities over the vast ranges encountered in nature.
- The three fundamental units are abbreviated as follows: meter, m; kilogram, kg; second, s. The metric system also uses a standard set of prefixes to denote each order of magnitude greater than or lesser than the fundamental unit itself.
- Unit conversions involve changing a value expressed in one type of unit to another type of unit. This is done by using conversion factors, which are ratios relating equal quantities of different units.
Conceptual Questions
1: Identify some advantages of metric units.
Problems & Exercises
1: The speed limit on some interstate highways is roughly 100 km/h. (a) What is this in meters per second? (b) How many miles per hour is this?
2: American football is played on a 100-yd-long field, excluding the end zones. How long is the field in meters? (Assume that 1 meter equals 1.094 yard)
3: What is the height in meters of a person who is 6 ft 1.0 in. tall? (Assume that 1 meter equals 39.37 in.)
4: Which of the following are fundamental units, which are derived units?
| Meter | Joule (kg*m2/s2) |
| meter per second | second |
| Newton (kg*m/s2) | g/cm^3 |
| cm2 | m3 |
Glossary
- physical quantity
- a characteristic or property of an object that can be measure or calculated from other measurements
- units
- a standard used for expressing and comparing measurements
- SI units
- the international system of units that scientist in most countries have agreed to use; includes units such as meters, liters, and grams
- English units
- system of measurement used in the United States; includes units of measurement such as feet, gallons, and pounds
- fundamental units
- units that can only be expressed relative to the procedure used to measure them
- derived units
- units that can be calculated using algebraic combinations of the fundamental units
- second
- the SI unit for time, abbreviated (s)
- meter
- the SI unit for length, abbreviated (m)
- kilogram
- the SI unit for mass, abbreviated (kg)
- metric system
- a system in which values can be calculated in factors of 10
- order of magnitude
- refers to the size of a quantity as it related to a power of 10
Solutions
Problems & Exercises
1: (a) 27.8 m/s b) 62.1 miles per hour or mph
2: 91.44m
3: 1.85m
4: Fundamental units: meter, second
Derived quantity: Joule, meter per second, g/cm3, Newton, cm2, m3
Media Attributions
- Mars Climate Orbiter

