The Structure of Crystalline Solids

Yu Kay Law

5 The Structure of Crystalline Solids

Purpose

To investigate the structures of various commonly found motifs in crystalline structures.

Learning Outcomes

Define unit cell and describe how repeating unit cells lead to crystalline solids.
Be able to describe the structures of the following crystal structures: simple cubic, body-centered cubic, face-centered cubic, hexagonal close packing, and sodium chloride.
Define and determine the coordination numbers for crystalline solids.
Perform calculations relating the density and edge length for simple cubic, body-centered cubic, and face-centered cubic unit cells.

Textbook Reference

Tro, Chemistry – A Molecular Approach (5th Ed), Ch. 13.3 and 13.5.

Introduction

Many solids are found in regularly packed arrays of atoms, molecules, or ions. These are crystalline structures which form regularly packed structures and therefore are found to have distinctive, fixed structures . As a result, beautiful crystals of various elements and compounds can be found, such as that of sodium chloride and even proteins such as insulin can be observed. The presence of such structures imply that regular conformations of such elements or compounds can be found, and these can be measured using techniques such as X-ray diffraction.

(a) a large crystal of sodium chloride; (b) space-grown crystals of insulin — Crystals of (a) sodium chloride and (b) insulin. Credit: (a) Hans-Joachim Engelhardt; (b) NASA.

The Unit Cell

A crystal structure is formed from a repeating array of identical units that are stacked in a repeating array. Each identical unit that can be stacked is referred to as a unit cell. Therefore, when classifying types of structures, what we typically do is to identify the structure of its unit cell.

A diagram of two images is shown. In the first image, a cube with a sphere at each corner is shown. The cube is labeled “Unit cell” and the spheres at the corners are labeled “Lattice points.” The second image shows the same cube, but this time it is one cube amongst eight that make up a larger cube. The original cube is shaded a color while the other cubes are not. — A unit cell shows the locations of lattice points repeating in all directions. Source: OpenStax Chemistry 2e

At the atomic level, a number of distinct common motifs for crystal structures can be observed. In this experiment, we will explore several common motifs for elemental crystal structures as well as the crystal structure of sodium chloride. We are also focusing on cubic unit cells.

Sharing Atoms Between Unit Cells

If we look at the face centered cubic unit cell, we will find that there is one atom at each corner, and one at the middle of each face.

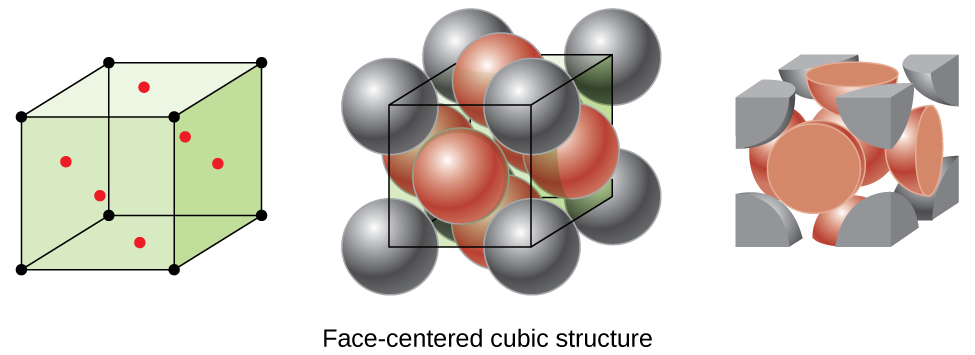
Three images are shown. The first image shows a cube with black dots at each corner and red dots in the center of each face of the cube while the second image is composed of eight spheres that are stacked together to form a cube with six more spheres, one located on each face of the structure. Dots at the center of each corner sphere are connected to form a cube shape. The name under this image reads “Face-centered cubic structure.” The third image is the same as the second, but only shows the portions of the spheres that lie inside the cube shape. — A face centered cubic unit cell. Source: OpenStax Chemistry 2e

If you look at this unit cell, only half of the atoms on each face (the green atoms) are present within the center of the unit cell we’re talking about; the other half is in the adjacent unit cell. Similarly, only 1/8 of the unit cell at each corner is inside the central unit cell. This is summarized in the table below:

Position	Proportion in a Unit Cell
center of unit cell/completely inside	1
on face of unit cell	1/2
on unit cell edge	1/4
at corner of unit cell	1/8

The Menagerie of Unit Cells

Each crystal structure has a number of distinctive properties distinguishing it from other crystal structures; in turn, many of these properties are shared between substances with the same unit cell structure. One of these properties is the coordination number of a given atom.

Coordination Number

The coordination number of a particular species in a unit cell is the number of other atoms with which a particular atom of that species is in direct contact.

Example

A simple cubic unit cell has one atom at each corner of the unit cell. In this case, the atoms along each edge touch each other. Therefore, each atom is in direct contact with six different atoms (one on each side – up, down, front, back, left, right) and hence the coordination number is six.

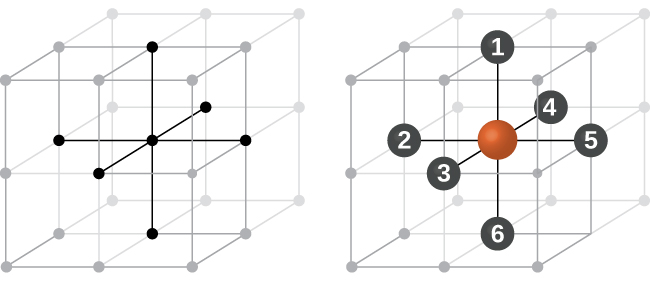
For fairly obvious reasons, for an ionic solid you would not expect ions with the same charge to be in direct contact with each other!

Visualization Exercise

In each of these cases, we will use an idealized view of the atoms and/or ions being perfect spheres. For these purposes this level of detail will be sufficient. In Chemistry, depending on the problem being studied, we apply different levels of simplifications in the models that we use. Choosing the right level of complexity is key to solving problems without running into unnecessary complexities while maintaining the essence of the chemistry.

In this exercise, you will study the crystal structures of a number of different (mostly metallic) elements as well as sodium chloride, an ionic solid. To do this, rather than trying to do this with paper-and-pencil type exercises or solid models, you will view a set of visualizations produced by Dean H. Johnstone at Otterbein College. Through viewing these structures, you will hopefully obtain a general understanding of a number of the main types of unit cell structures of crystalline solids.

Materials Studied

You will study a range of crystalline solid models as described below:

Simple Cubic, such as polonium.
Body-Centered Cubic, such as barium
Face-Centered Cubic (or cubic closest packed), such as copper.

We will also study the crystalline structure of sodium chloride.

Procedures

Here, we outline how to complete the first part of the exercise with the simple cubic (Po) structure. The rest of the structures can be studied in a similar manner.

Open the Crystals @ Otterbein crystal packing visualization.
Under “Select Example”, click on “simple cubic (Po)”. A number of command buttons can be found on the right hand side, while in the middle you will see a depiction of the crystal structure for Polonium in a big square Jmol box.

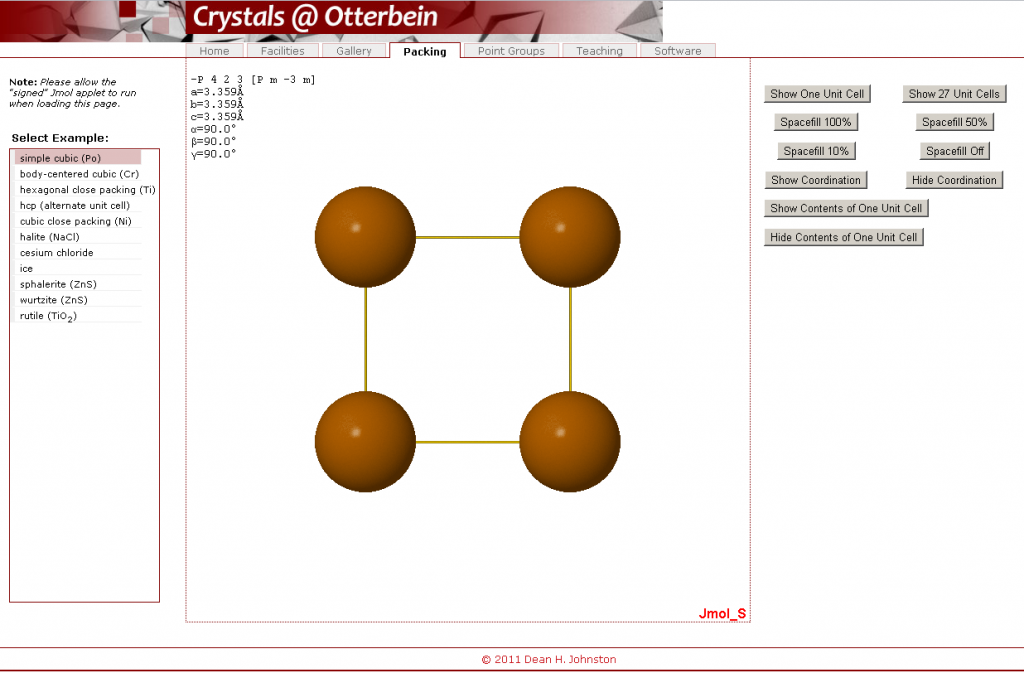
Screenshot of the crystals@otterbein site — Screenshot of the Crystals @ Otterbein website.

It may take a little trial and improvement to get the website to do what you want it to do, so please persevere a little. If you are having difficulties with this please don’t hesitate to get help.

In this interface, several different actions can be completed. You are not required to perform any specific action; rather, these are actions that may help you from time to time, and you can choose to do whatever it takes to solve your problem.

To rotate the molecule, click on the big square Jmol box containing the crystal structure and attempt to drag the molecule such that it rotates.
To zoom in or out of the crystal structure, press on the shift button and, simultaneously, move the mouse up or down while clicking down on the structure. A number of additional functions are available through the menu you can access by right-clicking on the molecule.
Show either a single unit cell or 27 unit cells (3 x 3 x 3) at once. Note that this shows all atoms as whole atoms even if only part of the atom is in each of these unit cells. In either case, it would help you determine the contents of the unit cells and how they, combined, form a crystal lattice.
Change the proportion of the actual volume of the atoms that is taken up by the depicted spheres (e.g. “Spacefill 100%” means that the atoms take up the volume that they do in the ideal crystal structure (with atoms touching each other where appropriate). Note, however, that often you can see more detail with a 50% spacefill.
You can choose whether you will see lines joining atoms that are touching each other by selecting “Show Coordination” or “Hide Coordination”. By counting these, you will be able to determine the coordination number for a given element by counting these links.
- When doing this, you must be careful to ensure that you are counting from a central atom (not on the edge of a unit cell); otherwise, you will undercount. Also, you will not be able to visualize this with a 100% spacefill setting
“Show Contents of One Unit Cell” would show a single unit cell with only the parts of each atom that are actually inside the unit cell shown. This can be undone by “Hide Contents of One Unit Cell”.
For some of the crystal structures, “Show layers” shows the closest parallel layers of atoms present in a given structure.

For a cubic unit cell, the proportion of an atom within a given unit cell is given above. from which the number of atoms in each unit cell can be determined. For this purpose, parts of different atoms can be added up to form a net of one (or more) atoms, as illustrated in the example below:

Examples

The ZnS (zincblende) crystal structure contains four zinc atoms completely inside each unit cell, while there are sulfur atoms at each corner and on each face of the unit cell:

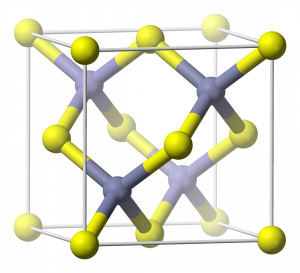
A zincblende unit cell. — A zincblende unit cell, with yellow sulfur atoms and purple zinc atoms.

Therefore, in each unit cell, there are:

$\displaystyle 4\mbox{ central atoms in unit cell} \times \frac{1\mbox{ atom}}{\mbox{u.c.}} = 4\mbox{ S atoms}$
$\displaymath 8 \mbox{ corners} \times \frac{\frac{1}{8} \mbox{atoms}}{\mbox{corner of u.c.}} + 6\mbox{ faces} \times \frac{\frac{1}{2} \mbox{atoms}}{\mbox{face of u.c.}} = 4 \mbox{ S atoms}$

Using similar techniques, you will learn to describe the various unit cells in this experiment. Examine each of the unit cells that are studied in this experiment carefully, and answer the questions on the report form.

Calculations Involving Unit Cells

Given the structure of the unit cell, we can relate the following quantities through calculations:

Density (which you can measure).
Unit cell length (which can be determined using X-ray crystallography).
Atomic radius.

For this section, we will focus only on cubic unit cells containing only one element, though the principles can be extended to more complex unit cells.

Relating the Density and Unit Cell Length

Given that we are focusing on cubic unit cells, the volume of a unit cell $V$ is related to the unit cell edge length $l$ by $V=l^3$ .

Space not occupied by any atom within a unit cell is still part of the volume of that unit cell.

We can also, following the examples above, determine the number of atoms in a given unit cell.

Recalling that the density of any substance is given by

(1) $\begin{equation*} d = \frac{m}{V} \end{equation*}$

The mass of a unit cell can be found by determining the mass of the atoms within a unit cell, using Avogadro’s number as needed. As mentioned above, the volume and edge length can be related relatively easily.

Examples

Sodium has a density of 0.971 g/cm³ and is known to be in a unit cell where there are two atoms per unit cell. We can start by finding the volume of one unit cell:

$\begin{align*} ? \textrm{ cm}^3 &= 1\textrm{ unit cell} \times \frac{2\textrm{ atoms}}{\textrm{unit cell}} \times \frac{1\textrm{ mol}}{6.022\times 10^{23}\textrm{ atoms}} \times \frac{22.99\textrm{ g}}{\textrm{mol}}\times \frac{1\mbox{ cm}^3}{0.971 \mbox{g}} \\ &= 7.86\times 10^{-23} \textrm{ g} \end{align*}$

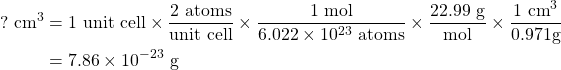
and then, by taking the cube root, we find that the edge length is

(2) $\begin{equation*}l = \sqrt[3]{7.86\times 10^{-23}} = 4.3\times 10^{-8}\textrm{ cm} = 4.3 \textrm{ \AA}\end{equation*}$

Relating the Edge Length and Atomic Radius

In this case, we need to recognize that for each unit cell, certain atoms can be deemed to be bordering each other, and hence a certain number of radii can be considered to be related to a characteristic length of the unit cell.