8 Differential Equations
A differential equation is one containing an independent variable, a function ![]() of that variable, and derivatives of
of that variable, and derivatives of ![]() [15]. What we are going to focus on is how to find the solutions of a differential equation. That is, we want to know what functions
[15]. What we are going to focus on is how to find the solutions of a differential equation. That is, we want to know what functions ![]() can be plugged into the differential equation and result in a true statement.
can be plugged into the differential equation and result in a true statement.
There are many different forms that a differential equation can take, and each form has a different method for finding solutions. For our purposes here, we are going to focus on the separable differential equation.
Larson and Edwards provide us with the process that is used to find the general solution of a separable differential equation [15]. Get all ![]() -terms and
-terms and ![]() on one side of the equation and the
on one side of the equation and the ![]() -terms and
-terms and ![]() on the other side. Then, integrate until the derivative terms are gone and simplify. This form is known as a separable equation because we are able to separate the
on the other side. Then, integrate until the derivative terms are gone and simplify. This form is known as a separable equation because we are able to separate the ![]() -terms and
-terms and ![]() from the
from the ![]() -terms and
-terms and ![]() .
.
Let’s now look at an example that I completed for this paper that comes from Larson and Edwards [15].
Find the general solution of
Solution
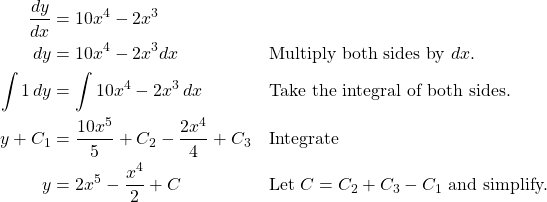
Therefore, the general solution of ![]() is
is
![]()
This is known as the general solution because we can substitute any real number in for ![]() , and the equation would still be a solution for the differential equation. Consequently, there are an infinite number of solutions represented by the general solution
, and the equation would still be a solution for the differential equation. Consequently, there are an infinite number of solutions represented by the general solution
![]()
The problem above was a very simple differential equation. All we had to do was integrate. However, in a Differential Equations course, the problems are more complicated and require much more than just simply integrating. But regardless of how challenging the problem is, if the general solution is found, it can be substituted into the original differential equation. Then, if both sides of the equal sign are in fact equal after differentiating and simplifying, we know that we have found the correct general solution.
